Stress (mechanics)
| Continuum mechanics | ||||||||||
|
||||||||||



 can be used
can be used
 is a reasonable approximation.[1]
is a reasonable approximation.[1]In continuum mechanics, stress is a measure of the intensity of the internal forces acting within a deformable body. Mathematically, it is a measure of the average force per unit area of a surface within a the body on which internal forces act. These internal forces are produced between the particles in the body as a reaction to external forces applied on the body. Because the loaded deformable body is assumed to behave as a continuum, these internal forces are distributed continuously within the volume of the material body, and result in deformation of the body's shape. Beyond certain limits of material strength, this can lead to a permanent change of shape or physical failure.
The SI unit for stress is the pascal (symbol Pa), which is equivalent to one newton (force) per square meter (unit area). The unit for stress is the same as that of pressure, which is also a measure of force per unit area.
Introduction
In continuum mechanics, stress is a measure of the average force per unit area of a surface within a deformable body on which internal forces act. It is a measure of the intensity of the internal forces acting between particles of a deformable body across imaginary internal surfaces[2]. These internal forces are produced between the particles in the body as a reaction to external forces applied on the body. External forces are either surface forces or body forces. Because the loaded deformable body is assumed to behave as a continuum, these internal forces are distributed continuously within the volume of the material body, i.e. the stress distribution in the body is expressed as a piecewise continuous function of space coordinates and time.
Normal and shear stresses
For the simple case of a body axially loaded, e.g. a prismatic bar subjected to tension or compression by a force passing through its centroid (Figures 1.2 and 1.3) the stress  , or intensity of internal forces, can be obtained by dividing the total normal force
, or intensity of internal forces, can be obtained by dividing the total normal force  , determined from the equilibrium of forces, by the cross-sectional area
, determined from the equilibrium of forces, by the cross-sectional area  of the prism it is acting upon. The normal force can be a tensile force if acting outward from the plane, or compressive force if acting inward to the plane. In the case of a prismatic bar axially loaded, the stress
of the prism it is acting upon. The normal force can be a tensile force if acting outward from the plane, or compressive force if acting inward to the plane. In the case of a prismatic bar axially loaded, the stress  is represented by a scalar called engineering stress or nominal stress that represents an average stress (
is represented by a scalar called engineering stress or nominal stress that represents an average stress ( ) over the area, meaning that the stress in the cross section is uniformly distributed. Thus, we have
) over the area, meaning that the stress in the cross section is uniformly distributed. Thus, we have
A different type of stress is obtained when transverse forces  are applied to the prismatic bar as shown in Figure 1.4. Considering the same cross-section as before, from static equilibrium the internal force has a magnitude equal to
are applied to the prismatic bar as shown in Figure 1.4. Considering the same cross-section as before, from static equilibrium the internal force has a magnitude equal to  and in opposite direction parallel to the cross-section.
and in opposite direction parallel to the cross-section.  is called the shear force. Dividing the shear force
is called the shear force. Dividing the shear force  by the area
by the area  of the cross section we obtain the shear stress. In this case the shear stress
of the cross section we obtain the shear stress. In this case the shear stress  is a scalar quantity representing an average shear stress (
is a scalar quantity representing an average shear stress ( ) in the section, i.e. the stress in the cross-section is uniformly distributed.
) in the section, i.e. the stress in the cross-section is uniformly distributed.
In Figure 1.3, the normal stress is observed in two planes  and
and  of the axially loaded prismatic bar. The stress on plane
of the axially loaded prismatic bar. The stress on plane  , which is closer to the point of application of the load
, which is closer to the point of application of the load  , varies more across the cross-section than that of plane
, varies more across the cross-section than that of plane  . However, if the cross-sectional area of the bar is very small, i.e. the bar is slender, the variation of stress across the area is small and the normal stress can be approximated by
. However, if the cross-sectional area of the bar is very small, i.e. the bar is slender, the variation of stress across the area is small and the normal stress can be approximated by  . On the other hand, the variation of shear stress across the section of a prismatic bar cannot be assumed to be uniform.
. On the other hand, the variation of shear stress across the section of a prismatic bar cannot be assumed to be uniform.
Stress modelling (Cauchy)
In general, stress is not uniformly distributed over the cross-section of a material body, and consequently the stress at a point in a given region is different from the average stress over the entire area. Therefore, it is necessary to define the stress not over a given area but at a specific point in the body (Figure 1.1). According to Cauchy, the stress at any point in an object assumed to behave as a continuum is completely defined by the nine components  of a second-order tensor of type (0,2) known as the Cauchy stress tensor,
of a second-order tensor of type (0,2) known as the Cauchy stress tensor,  :
:
The Cauchy stress tensor obeys the tensor transformation law under a change in the system of coordinates. A graphical representation of this transformation law is the Mohr’s circle for stress.
The Cauchy stress tensor is used for stress analysis of material bodies experiencing small deformations. For large deformations, also called finite deformations, other measures of stress, such as the first and second Piola-Kirchhoff stress tensors, the Biot stress tensor, and the Kirchhoff stress tensor, are required.
According to the principle of conservation of linear momentum, if a continuous body is in static equilibrium it can be demonstrated that the components of the Cauchy stress tensor in every material point in the body satisfy the equilibrium equations (Cauchy’s equations of motion for zero acceleration). At the same time, according to the principle of conservation of angular momentum, equilibrium requires that the summation of moments with respect to an arbitrary point is zero, which leads to the conclusion that the stress tensor is symmetric, thus having only six independent stress components instead of the original nine.
There are certain invariants associated with the stress tensor, whose values do not depend upon the coordinate system chosen or the area element upon which the stress tensor operates. These are the three eigenvalues of the stress tensor, which are called the principal stresses. Solids, liquids, and gases have stress fields. Static fluids support normal stress but will flow under shear stress. Moving viscous fluids can support shear stress (dynamic pressure). Solids can support both shear and normal stress, with ductile materials failing under shear and brittle materials failing under normal stress. All materials have temperature dependent variations in stress-related properties, and non-Newtonian materials have rate-dependent variations.
Stress analysis
The determination of the internal distribution of stresses, viz stress analysis, is required in engineering, for the study and design of structures, e.g. tunnels, dams, mechanical parts, and structural frames, among others, under prescribed or expected loads. To determine the distribution of stress in the structure it is necessary to solve a boundary-value problem by specifying the boundary conditions, i.e. displacements and/or forces on the boundary. Constitutive equations, such as Hooke’s Law for linear elastic materials, are used to describe the stress:strain relationship in these calculations. A boundary-value problem based on the theory of elasticity is applied to structures expected to deform elastically, with infinitesimal strains, under design loads. When the loads applied to the structure induce plastic deformations, the theory of plasticity is implemented.
The stress analysis can be simplified in cases where the physical dimensions and the distribution of loads allow the structure to be treated as one-dimensional or two-dimensional. For a two-dimensional analysis a plane stress or a plane strain condition can be assumed.
Alternatively, experimental determination of stresses can be carried out using the photoelastic method.
Approximate solutions for boundary-value problems can be obtained through the use of numerical methods such as the Finite Element Method, the Finite Difference Method, and the Boundary Element Method, which are implemented in computer programs. Analytical or closed-form solutions can be obtained for simple geometries, constitutive relations, and boundary conditions.
In designing structures, calculated stresses are restricted to be less than an specified allowable stress, also known as working or designed stress. Allowable stress is chosen as some fraction of the yield strength or of the ultimate strength of the material of which the structure is made. The ratio of the ultimate stress to the allowable stress is defined as the factor of safety. Laboratory tests are usually performed on material samples in order to determine the yield strength and the ultimate strength that the material can withstand before failure.
Engineering quantities are usually measured in megapascals (MPa) or gigapascals (GPa). In imperial units, stress is expressed in pounds-force per square inch (psi) or kilopounds-force per square inch (ksi).
Forces in a continuum
Continuum mechanics deals with deformable bodies, as opposed to rigid bodies. A solid is a deformable body that possesses shear strength, sc. a solid can support shear forces (forces parallel to the material surface on which they act). Fluids, on the other hand, do not sustain shear forces. For the study of the mechanical behavior of solids and fluids these are assumed to be continuous bodies, which means that the matter fills the entire region of space it occupies, despite the fact that matter is made of atoms, has voids, and is discrete. Therefore, when continuum mechanics refers to a point or particle in a continuous body it does not describe a point in the interatomic space or an atomic particle, rather an idealized part of the body occupying that point.
Following the classical dynamics of Newton and Euler, the motion of a material body is produced by the action of externally applied forces which are assumed to be of two kinds: surface forces  and body forces
and body forces  .[3] Thus, the total force
.[3] Thus, the total force  applied to a body or to a portion of the body can be expressed as:
applied to a body or to a portion of the body can be expressed as:
Surface forces or contact forces, expressed as force per unit area, can act either on the bounding surface of the body, as a result of mechanical contact with other bodies, or on imaginary internal surfaces that bound portions of the body, as a result of the mechanical interaction between the parts of the body to either side of the surface (Euler-Cauchy's stress principle). When a body is acted upon by external contact forces, internal contact forces are then transmitted from point to point inside the body to balance their action, according to Newton's second law of motion of conservation of linear momentum and angular momentum (for continuous bodies these laws are called the Euler's equations of motion). The internal contact forces are related to the body's deformation through constitutive equations. This article is concerned with the manner in which internal contact forces are mathematically described and how they relate to the motion of the body, independent of the body's material makeup.[4]
The distribution of internal contact forces throughout the volume of the body is assumed to be continuous. Therefore, there exists a contact force density or Cauchy traction field [3]  that represents this distribution in a particular configuration of the body at a given time
that represents this distribution in a particular configuration of the body at a given time  . It is not a vector field because it depends not only on the position
. It is not a vector field because it depends not only on the position  of a particular material point, but also on the local orientation of the surface element as defined by its normal vector
of a particular material point, but also on the local orientation of the surface element as defined by its normal vector  [5].
[5].
Any differential area  with normal vector
with normal vector  of a given internal surface area
of a given internal surface area  , bounding a portion of the body, experiences a contact force
, bounding a portion of the body, experiences a contact force  arising from the contact between both portions of the body on each side of
arising from the contact between both portions of the body on each side of  , and it is given by
, and it is given by
where  is the surface traction,[6] also called stress vector,[7] traction,[8] or traction vector,[9]. The stress vector is a frame-indifferent vector. The stress vector will be explained in more detail later (Euler-Cauchy's stress principle).
is the surface traction,[6] also called stress vector,[7] traction,[8] or traction vector,[9]. The stress vector is a frame-indifferent vector. The stress vector will be explained in more detail later (Euler-Cauchy's stress principle).
The total contact force on the particular internal surface  is then expressed as the sum (surface integral) of the contact forces on all differential surfaces
is then expressed as the sum (surface integral) of the contact forces on all differential surfaces  :
:
The concept of stress can then be thought as a measure of the intensity of the internal contact forces acting between particles of the body across imaginary internal surfaces.[2] In other words, stress is a measure of the average quantity of force exerted per unit area of the surface on which these internal forces act. The intensity of contact forces is related, specifically in an inverse proportion, to the area of contact. For example, if we compare a force applied to a small area and a distributed load of the same resultant magnitude applied to a larger area, we find that the effects or intensities of these two forces are locally different because the stresses are not the same.
In continuum mechanics a body is considered stress-free if the only forces present are those inter-atomic forces (ionic, metallic, and van der Waals forces) required to hold the body together and to keep its shape in the absence of all external influences, including gravitational attraction.[9][10] Stresses generated during manufacture of the body to a specific configuration are also excluded when considering stresses in a body. Therefore, the stresses considered in continuum mechanics are only those produced by deformation of the body, sc. only relative changes in stress are considered, not the absolute values of stress.
Body forces are forces originating from sources outside of the body[11] that act on the volume (or mass) of the body. Saying that body forces are due to outside sources implies that the interaction between different parts of the body (internal forces) are manifested through the contact forces alone.[6] These forces arise from the presence of the body in force fields, e.g. gravitational field (gravitational forces) or electromagnetic field (electromagnetic forces), or from inertial forces when bodies are in motion. As the mass of a continuous body is assumed to be continuously distributed, any force originating from the mass is also continuously distributed. Thus, body forces are specified by vector fields which are assumed to be continuous over the entire volume of the body,[12] i.e. acting on every point in it. Body forces are represented by a body force density  (per unit of mass), which is a frame-indifferent vector field.
(per unit of mass), which is a frame-indifferent vector field.
In the case of gravitational forces, the intensity of the force depends on, or is proportional to, the mass density  of the material, and it is specified in terms of force per unit mass (
of the material, and it is specified in terms of force per unit mass ( ) or per unit volume (
) or per unit volume ( ). These two specifications are related through the material density by the equation
). These two specifications are related through the material density by the equation  . Similarly, the intensity of electromagnetic forces depends upon the strength (electric charge) of the electromagnetic field.
. Similarly, the intensity of electromagnetic forces depends upon the strength (electric charge) of the electromagnetic field.
The total body force applied to a continuous body is expressed as
Body forces and contact forces acting on the body lead to corresponding moments of force (torques) relative to a given point. Thus, the total applied torque  about the origin is given by
about the origin is given by
In certain situations, not commonly considered in the analysis of the mechanical behavior or materials, it becomes necessary to include two other types of forces: these are body moments and couple stresses[13][14] (surface couples,[11] contact torques[12]). Body moments, or body couples, are moments per unit volume or per unit mass applied to the volume of the body. Couple stresses are moments per unit area applied on a surface. Both are important in the analysis of stress for a polarized dielectric solid under the action of an electric field, materials where the molecular structure is taken into consideration (e.g. bones), solids under the action of an external magnetic field, and the dislocation theory of metals.[7][8][11]
Materials that exhibit body couples and couple stresses in addition to moments produced exclusively by forces are called polar materials.[8][12] Non-polar materials are then those materials with only moments of forces. In the classical branches of continuum mechanics the development of the theory of stresses is based on non-polar materials.
Thus, the sum of all applied forces and torques (with respect to the origin of the coordinate system) in the body can be given by
Euler's laws of motion for a continuum
The density of internal forces at every point in a deformable body are not necessarily equal, i.e. there is a distribution of stresses throughout the body. This variation of internal forces throughout the body is governed by Newton's second law of motion of conservation of linear momentum and angular momentum, which normally are applied to a mass particle but are extended in continuum mechanics to a body of continuously distributed mass. For continuous bodies these laws are called Euler’s equations of motion. If a body is represented as an assemblage of discrete particles, each governed by Newton’s laws of motion, then Euler’s equations can be derived from Newton’s laws. Euler’s equations can, however, be taken as axioms describing the laws of motion for extended bodies, independently of any particle structure.[5]
Let the coordinate system  be an inertial frame of reference. Let
be an inertial frame of reference. Let  be the position vector of a particle or point
be the position vector of a particle or point  in the continuous body with respect to the origin of the coordinate system, and
in the continuous body with respect to the origin of the coordinate system, and  the velocity vector of point
the velocity vector of point  .
.
Euler’s first axiom or law (law of balance of linear momentum or balance of forces) states that in an inertial frame the time rate of change of linear momentum  of an arbitrary portion of a continuous body is equal to the total applied force
of an arbitrary portion of a continuous body is equal to the total applied force  acting on the considered portion, and it is expressed as
acting on the considered portion, and it is expressed as
Euler’s second axiom or law (law of balance of angular momentum or balance of torques) states that in an inertial frame the time rate of change of angular momentum  of an arbitrary portion of a continuous body is equal to the total applied torque
of an arbitrary portion of a continuous body is equal to the total applied torque  acting on the considered portion, and it is expressed as
acting on the considered portion, and it is expressed as
The derivatives of  and
and  are material derivatives.
are material derivatives.
Euler-Cauchy stress principle

 of the internal surface
of the internal surface  in a continuum, as a result of the interaction between the two portions of the continuum separated by the surface
in a continuum, as a result of the interaction between the two portions of the continuum separated by the surface
 of the internal surface
of the internal surface  in a continuum, as a result of the interaction between the two portions of the continuum separated by the surface
in a continuum, as a result of the interaction between the two portions of the continuum separated by the surface
 , and can be resolved into two components: one component normal to the plane, called normal stress
, and can be resolved into two components: one component normal to the plane, called normal stress  , and another component parallel to this plane, called the shearing stress
, and another component parallel to this plane, called the shearing stress  .
.The Euler-Cauchy stress principle states that upon any surface (real or imaginary) that divides the body, the action of one part of the body on the other is equivalent (equipollent) to the system of distributed forces and couples on the surface dividing the body,[15] and it is represented by a vector field  , called the stress vector, defined on the surface
, called the stress vector, defined on the surface  and assumed to depend continuously on the surfaces unit vectors
and assumed to depend continuously on the surfaces unit vectors  .[8][12]
.[8][12]
To explain this principle, we consider an imaginary surface  passing through an internal material point
passing through an internal material point  dividing the continuous body into two segments, as seen in Figure 2.1a or 2.1b (some authors use the cutting plane diagram and others use the diagram with the arbitrary volume inside the continuum enclosed by the surface
dividing the continuous body into two segments, as seen in Figure 2.1a or 2.1b (some authors use the cutting plane diagram and others use the diagram with the arbitrary volume inside the continuum enclosed by the surface  ). The body is subjected to external surface forces
). The body is subjected to external surface forces  and body forces
and body forces  . The internal contact forces being transmitted from one segment to the other through the dividing plane, due to the action of one portion of the continuum onto the other, generate a force distribution on a small area
. The internal contact forces being transmitted from one segment to the other through the dividing plane, due to the action of one portion of the continuum onto the other, generate a force distribution on a small area  , with a normal unit vector
, with a normal unit vector  , on the dividing plane
, on the dividing plane  . The force distribution is equipollent to a contact force
. The force distribution is equipollent to a contact force  and a couple stress
and a couple stress  , as shown in Figure 2.1a and 2.1b. Cauchy’s stress principle asserts[9] that as
, as shown in Figure 2.1a and 2.1b. Cauchy’s stress principle asserts[9] that as  becomes very small and tends to zero the ratio
becomes very small and tends to zero the ratio  becomes
becomes  and the couple stress vector
and the couple stress vector  vanishes. In specific fields of continuum mechanics the couple stress is assumed not to vanish; however, as stated previously, in classical branches of continuum mechanics we deal with non-polar materials which do not consider couple stresses and body moments. The resultant vector
vanishes. In specific fields of continuum mechanics the couple stress is assumed not to vanish; however, as stated previously, in classical branches of continuum mechanics we deal with non-polar materials which do not consider couple stresses and body moments. The resultant vector  is defined as the stress vector or traction vector given by
is defined as the stress vector or traction vector given by  at point
at point  associated with a plane with a normal vector
associated with a plane with a normal vector  :
:
This equation means that the stress vector depends on its location in the body and the orientation of the plane on which it is acting.
Depending on the orientation of the plane under consideration, the stress vector may not necessarily be perpendicular to that plane, i.e. parallel to  , and can be resolved into two components:
, and can be resolved into two components:
- one normal to the plane, called normal stress

where  is the normal component of the force
is the normal component of the force  to the differential area
to the differential area 
- and the other parallel to this plane, called the shearing stress
 .
.
where 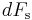 is the tangential component of the force
is the tangential component of the force  to the differential surface area
to the differential surface area  . The shear stress can be further decomposed into two mutually perpendicular vectors.
. The shear stress can be further decomposed into two mutually perpendicular vectors.
Cauchy’s postulate
According to the Cauchy Postulate, the stress vector  remains unchanged for all surfaces passing through a point
remains unchanged for all surfaces passing through a point  and having the same normal vector
and having the same normal vector  at
at  ,[6][16] i.e. having a common tangent at
,[6][16] i.e. having a common tangent at  . This means that the stress vector is only a function of the normal vector
. This means that the stress vector is only a function of the normal vector  , and it is not influenced by the curvature of the internal surfaces.
, and it is not influenced by the curvature of the internal surfaces.
Cauchy’s fundamental lemma
A consequence of Cauchy’s postulate is Cauchy’s Fundamental Lemma[6][10][11], also called the Cauchy reciprocal theorem[17], which states that the stress vectors acting on opposite sides of the same surface are equal in magnitude and opposite in direction. Cauchy’s fundamental lemma is equivalent to Newton's third law of motion of action and reaction, and it is expressed as
-
Derivation of Cauchy’s Lemma Derivation coming soon
Cauchy’s stress theorem – stress tensor
The state of stress at a point in the body is then defined by all the stress vectors  associated with all planes (infinite in number) that pass through that point [2]. However, according to Cauchy’s fundamental theorem [10], also called Cauchy’s stress theorem [11], merely by knowing the stress vectors on three mutually perpendicular planes, the stress vector on any other plane passing through that point can be found through coordinate transformation equations.
associated with all planes (infinite in number) that pass through that point [2]. However, according to Cauchy’s fundamental theorem [10], also called Cauchy’s stress theorem [11], merely by knowing the stress vectors on three mutually perpendicular planes, the stress vector on any other plane passing through that point can be found through coordinate transformation equations.
Cauchy’s stress theorem states that there exists a second-order tensor field  , called the Cauchy stress tensor, independent of
, called the Cauchy stress tensor, independent of  such that
such that  is a linear function of
is a linear function of  :
:
This equation implies that the stress vector  at any point
at any point  in a continuum associated with a plane with normal vector
in a continuum associated with a plane with normal vector  can be expressed as a function of the stress vectors on the planes perpendicular to the coordinate axes, i.e. in terms of the components
can be expressed as a function of the stress vectors on the planes perpendicular to the coordinate axes, i.e. in terms of the components  of the stress tensor
of the stress tensor  .
.
To prove this expression, consider a tetrahedron with three faces oriented in the coordinate planes, and with an infinitesimal area  oriented in an arbitrary direction specified by a normal vector
oriented in an arbitrary direction specified by a normal vector  (Figure 2.2). The tetrahedron is formed by slicing the infinitesimal element along an arbitrary plane
(Figure 2.2). The tetrahedron is formed by slicing the infinitesimal element along an arbitrary plane  . The stress vector on this plane is denoted by
. The stress vector on this plane is denoted by  . The stress vectors acting on the faces of the tetrahedron are denoted as
. The stress vectors acting on the faces of the tetrahedron are denoted as  ,
,  , and
, and  , and are by definition the components of the stress tensor
, and are by definition the components of the stress tensor  . This tetrahedron is sometimes called the Cauchy tetrahedron. From equilibrium of forces, i.e. Euler’s first law of motion (Newton’s second law of motion), we have
. This tetrahedron is sometimes called the Cauchy tetrahedron. From equilibrium of forces, i.e. Euler’s first law of motion (Newton’s second law of motion), we have

A note on the sign convention: The tetrahedron is formed by slicing a parallelepiped along an arbitrary plane n. So, the force acting on the plane n is the reaction exerted by the other half of the parallelepiped and has an opposite sign.
where the right-hand-side of the equation represents the product of the mass enclosed by the tetrahedron and its acceleration:  is the density,
is the density,  is the acceleration, and
is the acceleration, and  is the height of the tetrahedron, considering the plane
is the height of the tetrahedron, considering the plane  as the base. The area of the faces of the tetrahedron perpendicular to the axes can be found by projecting
as the base. The area of the faces of the tetrahedron perpendicular to the axes can be found by projecting  into each face (using the dot product):
into each face (using the dot product):
and then can be substituted into the equation to cancel out  :
:
To consider the limiting case as the tetrahedron shrinks to a point, 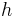 must go to 0 (intuitively, plane
must go to 0 (intuitively, plane  is translated along
is translated along  towards
towards  ). As a result, the right-hand-side of the equation approaches 0, thus
). As a result, the right-hand-side of the equation approaches 0, thus

Assuming a material element (Figure 2.3) with planes perpendicular to the coordinate axes of a Cartesian coordinate system, the stress vectors associated with each of the element planes, i.e.  ,
,  , and
, and  can be decomposed into a normal component and two shear components, i.e. components in the direction of the three coordinate axes. For the particular case of a surface with normal unit vector oriented in the direction of the
can be decomposed into a normal component and two shear components, i.e. components in the direction of the three coordinate axes. For the particular case of a surface with normal unit vector oriented in the direction of the  -axis, the normal stress is denoted by
-axis, the normal stress is denoted by  , and the two shear stresses are denoted as
, and the two shear stresses are denoted as  and
and  :
:
In index notation this is
The nine components  of the stress vectors are the components of a second-order Cartesian tensor called the Cauchy stress tensor, which completely defines the state of stresses at a point and is given by
of the stress vectors are the components of a second-order Cartesian tensor called the Cauchy stress tensor, which completely defines the state of stresses at a point and is given by
where
 ,
,  , and
, and  are normal stresses, and
are normal stresses, and
 ,
,  ,
,  ,
,  ,
,  , and
, and  are shear stresses.
are shear stresses.
The first index  indicates that the stress acts on a plane normal to the
indicates that the stress acts on a plane normal to the  axis, and the second index
axis, and the second index  denotes the direction in which the stress acts. A stress component is positive if it acts in the positive direction of the coordinate axes, and if the plane where it acts has an outward normal vector pointing in the positive coordinate direction.
denotes the direction in which the stress acts. A stress component is positive if it acts in the positive direction of the coordinate axes, and if the plane where it acts has an outward normal vector pointing in the positive coordinate direction.
The Voigt notation representation of the Cauchy stress tensor takes advantage of the symmetry of the stress tensor to express the stress as a six-dimensional vector of the form:
The Voigt notation is used extensively in representing stress-strain relations in solid mechanics and for computational efficiency in numerical structural mechanics software.
Thus, using the components of the stress tensor
or, equivalently,
Alternatively, in matrix form we have
Transformation rule of the stress tensor
It can be shown that the stress tensor is a contravariant second order tensor, which is a statement of how it transforms under a change of the coordinate system. From an  system to an
system to an  system, the components
system, the components  in the initial system are transformed into the components
in the initial system are transformed into the components  in the new system according to the tensor transformation rule (Figure 2.4):
in the new system according to the tensor transformation rule (Figure 2.4):
where  is the rotation matrix with components
is the rotation matrix with components  . In matrix form this is
. In matrix form this is

Expanding the matrix operation, and simplifying some terms by taking advantage of the symmetry of the stress tensor, gives:
A graphical representation of this transformation of stresses, for a two-dimensional (plane stress and plane strain) and a general three-dimensional state of stresses, is the Mohr's circle for stresses
Normal and shear stresses
The magnitude of the normal stress component,  , of any stress vector
, of any stress vector  acting on an arbitrary plane with normal vector
acting on an arbitrary plane with normal vector  at a given point in terms of the component of the stress tensor
at a given point in terms of the component of the stress tensor  is the dot product of the stress vector and the normal vector, thus
is the dot product of the stress vector and the normal vector, thus
The magnitude of the shear stress component,  , acting in the plane formed by the two vectors
, acting in the plane formed by the two vectors  and
and  , can then be found using the Pythagorean theorem, thus
, can then be found using the Pythagorean theorem, thus
where 
Equilibrium equations and symmetry of the stress tensor

When a body is in equilibrium the components of the stress tensor in every point of the body satisfy the equilibrium equations,
For example, for a hydrostatic fluid in equilibrium conditions, the stress tensor takes on the form:
 ,
,
where  is the hydrostatic pressure, and
is the hydrostatic pressure, and  is the kronecker delta.
is the kronecker delta.
-
Derivation of equilibrium equations Consider a continuum body (see Figure 4) occupying a volume  , having a surface area
, having a surface area  , with defined traction or surface forces
, with defined traction or surface forces  per unit area acting on every point of the body surface, and body forces
per unit area acting on every point of the body surface, and body forces  per unit of volume on every point within the volume
per unit of volume on every point within the volume  . Thus, if the body is in equilibrium the resultant force acting on the volume is zero, thus:
. Thus, if the body is in equilibrium the resultant force acting on the volume is zero, thus:
By definition the stress vector is
 , then
, thenUsing the Gauss's divergence theorem to convert a surface integral to a volume integral gives
For an arbitrary volume the integral vanishes, and we have the equilibrium equations
At the same time, equilibrium requires that the summation of moments with respect to an arbitrary point is zero, which leads to the conclusion that the stress tensor is symmetric, i.e.
-
Derivation of symmetry of the stress tensor Summing moments about point O (Figure 4) the resultant moment is zero as the body is in equilibrium. Thus, where
 is the position vector and is expressed as
is the position vector and is expressed asKnowing that
 and using Gauss's divergence theorem to change from a surface integral to a volume integral, we have
and using Gauss's divergence theorem to change from a surface integral to a volume integral, we haveThe second integral is zero as it contains the equilibrium equations. This leaves the first integral, where
 , therefore
, thereforeFor an arbitrary volume V, we then have
which is satisfied at every point within the body. Expanding this equation we have
 ,
,  , and
, and 
or in general
This proves that the stress tensor is symmetric
However, in the presence of couple-stresses, i.e. moments per unit volume, the stress tensor is non-symmetric. This also is the case when the Knudsen number is close to one,  , or the continuum is a Non-Newtonian fluid, which can lead to rotationally non-invariant fluids, such as polymers.
, or the continuum is a Non-Newtonian fluid, which can lead to rotationally non-invariant fluids, such as polymers.
Principal stresses and stress invariants
At every point in a stressed body there are at least three planes, called principal planes, with normal vectors  , called principal directions, where the corresponding stress vector is perpendicular to the plane, i.e., parallel or in the same direction as the normal vector
, called principal directions, where the corresponding stress vector is perpendicular to the plane, i.e., parallel or in the same direction as the normal vector  , and where there are no normal shear stresses
, and where there are no normal shear stresses  . The three stresses normal to these principal planes are called principal stresses.
. The three stresses normal to these principal planes are called principal stresses.
The components  of the stress tensor depend on the orientation of the coordinate system at the point under consideration. However, the stress tensor itself is a physical quantity and as such, it is independent of the coordinate system chosen to represent it. There are certain invariants associated with every tensor which are also independent of the coordinate system. For example, a vector is a simple tensor of rank one. In three dimensions, it has three components. The value of these components will depend on the coordinate system chosen to represent the vector, but the length of the vector is a physical quantity (a scalar) and is independent of the coordinate system chosen to represent the vector. Similarly, every second rank tensor (such as the stress and the strain tensors) has three independent invariant quantities associated with it. One set of such invariants are the principal stresses of the stress tensor, which are just the eigenvalues of the stress tensor. Their direction vectors are the principal directions or eigenvectors.
of the stress tensor depend on the orientation of the coordinate system at the point under consideration. However, the stress tensor itself is a physical quantity and as such, it is independent of the coordinate system chosen to represent it. There are certain invariants associated with every tensor which are also independent of the coordinate system. For example, a vector is a simple tensor of rank one. In three dimensions, it has three components. The value of these components will depend on the coordinate system chosen to represent the vector, but the length of the vector is a physical quantity (a scalar) and is independent of the coordinate system chosen to represent the vector. Similarly, every second rank tensor (such as the stress and the strain tensors) has three independent invariant quantities associated with it. One set of such invariants are the principal stresses of the stress tensor, which are just the eigenvalues of the stress tensor. Their direction vectors are the principal directions or eigenvectors.
A stress vector parallel to the normal vector  is given by:
is given by:
where  is a constant of proportionality, and in this particular case corresponds to the magnitudes
is a constant of proportionality, and in this particular case corresponds to the magnitudes  of the normal stress vectors or principal stresses.
of the normal stress vectors or principal stresses.
Knowing that  and
and  , we have
, we have
This is a homogeneous system, i.e. equal to zero, of three linear equations where  are the unknowns. To obtain a nontrivial (non-zero) solution for
are the unknowns. To obtain a nontrivial (non-zero) solution for  , the determinant matrix of the coefficients must be equal to zero, i.e. the system is singular. Thus,
, the determinant matrix of the coefficients must be equal to zero, i.e. the system is singular. Thus,
Expanding the determinant leads to the characteristic equation
where
The characteristic equation has three real roots  , i.e. not imaginary due to the symmetry of the stress tensor. The three roots
, i.e. not imaginary due to the symmetry of the stress tensor. The three roots  ,
,  , and
, and  are the eigenvalues or principal stresses, and they are the roots of the Cayley–Hamilton theorem. The principal stresses are unique for a given stress tensor. Therefore, from the characteristic equation it is seen that the coefficients
are the eigenvalues or principal stresses, and they are the roots of the Cayley–Hamilton theorem. The principal stresses are unique for a given stress tensor. Therefore, from the characteristic equation it is seen that the coefficients  ,
,  and
and  , called the first, second, and third stress invariants, respectively, have always the same value regardless of the orientation of the coordinate system chosen.
, called the first, second, and third stress invariants, respectively, have always the same value regardless of the orientation of the coordinate system chosen.
For each eigenvalue, there is a non-trivial solution for  in the equation
in the equation  . These solutions are the principal directions or eigenvectors defining the plane where the principal stresses act. The principal stresses and principal directions characterize the stress at a point and are independent of the orientation of the coordinate system.
. These solutions are the principal directions or eigenvectors defining the plane where the principal stresses act. The principal stresses and principal directions characterize the stress at a point and are independent of the orientation of the coordinate system.
If we choose a coordinate system with axes oriented to the principal directions, then the normal stresses will be the principal stresses and the stress tensor is represented by a diagonal matrix:
The principal stresses may be combined to form the stress invariants,  ,
,  , and
, and  .The first and third invariant are the trace and determinant respectively, of the stress tensor. Thus,
.The first and third invariant are the trace and determinant respectively, of the stress tensor. Thus,
Because of its simplicity, working and thinking in the principal coordinate system is often very useful when considering the state of the elastic medium at a particular point.
Maximum and minimum shear stresses
The maximum shear stress or maximum principal shear stress is equal to one-half the difference between the largest and smallest principal stresses, and acts on the plane that bisects the angle between the directions of the largest and smallest principal stresses, i.e. the plane of the maximum shear stress is oriented  from the principal stress planes. The maximum shear stress is expressed as
from the principal stress planes. The maximum shear stress is expressed as
Assuming  then
then
The normal stress component acting on the plane for the maximum shear stress is non-zero and it is equal to

-
Derivation of the maximum and minimum shear stresses [2][7][10][18][19][20][21] The normal stress can be written in terms of principal stresses  as
as
Knowing that
 , the shear stress in terms of principal stresses components is expressed as
, the shear stress in terms of principal stresses components is expressed asThe maximum shear stress at a point in a continuum body is determined by maximizing
 subject to the condition that
subject to the condition thatThis is a constrained maximization problem, which can be solved using the Lagrangian multiplier technique to convert the problem into an unconstrained optimization problem. Thus, the stationary values (maximum and minimum values)of
 occur where the gradient of
occur where the gradient of  is parallel to the gradient of
is parallel to the gradient of  .
.The Lagrangian function for this problem can be written as
where
 is the Lagrangian multiplier (which is different from the
is the Lagrangian multiplier (which is different from the  use to denote eigenvalues).
use to denote eigenvalues).The extreme values of these functions are
thence
These three equations together with the condition
 may be solved for
may be solved for  and
and 
By multiplying the first three equations by
 and
and  , respectively, and knowing that
, respectively, and knowing that  we obtain
we obtainAdding these three equations we get
this result can be substituted into each of the first three equations to obtain
Doing the same for the other two equations we have
A first approach to solve these last three equations is to consider the trivial solution
 . However this options does not fulfill the constrain
. However this options does not fulfill the constrain  .
.Considering the solution where
 and
and  , it is determine from the condition
, it is determine from the condition  that
that  , then from the original equation for
, then from the original equation for  it is seen that
it is seen that  . The other two possible values for
. The other two possible values for  can be obtained similarly by assuming
can be obtained similarly by assuming and
and 
 and
and 
Thus, one set of solutions for these four equations is:
These correspond to minimum values for
 and verifies that there are no shear stresses on planes normal to the principal directions of stress, as shown previously.
and verifies that there are no shear stresses on planes normal to the principal directions of stress, as shown previously.A second set of solutions is obtained by assuming
 and
and  . Thus we have
. Thus we haveTo find the values for
 and
and  we first add these two equations
we first add these two equationsKnowing that for

and
we have
and solving for
 we have
we haveThen solving for
 we have
we haveand
The other two possible values for
 can be obtained similarly by assuming
can be obtained similarly by assuming and
and 
 and
and 
Therefore the second set of solutions for
 , representing a maximum for
, representing a maximum for  is
isTherefore, assuming
 , the maximum shear stress is expressed by
, the maximum shear stress is expressed byand it can be stated as being equal to one-half the difference between the largest and smallest principal stresses, acting on the plane that bisects the angle between the directions of the largest and smallest principal stresses.
Stress deviator tensor
The stress tensor  can be expressed as the sum of two other stress tensors:
can be expressed as the sum of two other stress tensors:
- a mean hydrostatic stress tensor or volumetric stress tensor or mean normal stress tensor,
 , which tends to change the volume of the stressed body; and
, which tends to change the volume of the stressed body; and - a deviatoric component called the stress deviator tensor,
 , which tends to distort it.
, which tends to distort it.
where  is the mean stress given by
is the mean stress given by
The deviatoric stress tensor can be obtained by subtracting the hydrostatic stress tensor from the stress tensor:
Invariants of the stress deviator tensor
As it is a second order tensor, the stress deviator tensor also has a set of invariants, which can be obtained using the same procedure used to calculate the invariants of the stress tensor. It can be shown that the principal directions of the stress deviator tensor  are the same as the principal directions of the stress tensor
are the same as the principal directions of the stress tensor  . Thus, the characteristic equation is
. Thus, the characteristic equation is
where  ,
,  and
and  are the first, second, and third deviatoric stress invariants, respectively. Their values are the same (invariant) regardless of the orientation of the coordinate system chosen. These deviatoric stress invariants can be expressed as a function of the components of
are the first, second, and third deviatoric stress invariants, respectively. Their values are the same (invariant) regardless of the orientation of the coordinate system chosen. These deviatoric stress invariants can be expressed as a function of the components of  or its principal values
or its principal values  ,
,  , and
, and  , or alternatively, as a function of
, or alternatively, as a function of  or its principal values
or its principal values  ,
,  , and
, and  . Thus,
. Thus,
Because  , the stress deviator tensor is in a state of pure shear.
, the stress deviator tensor is in a state of pure shear.
A quantity called the equivalent stress or von Mises stress is commonly used in solid mechanics. The equivalent stress is defined as
Octahedral stresses

Considering the principal directions as the coordinate axes, a plane whose normal vector makes equal angles with each of the principal axes (i.e. having direction cosines equal to  ) is called an octahedral plane. There are a total of eight octahedral planes (Figure 6). The normal and shear components of the stress tensor on these planes are called octahedral normal stress
) is called an octahedral plane. There are a total of eight octahedral planes (Figure 6). The normal and shear components of the stress tensor on these planes are called octahedral normal stress  and octahedral shear stress
and octahedral shear stress  , respectively.
, respectively.
Knowing that the stress tensor of point O (Figure 6) in the principal axes is
the stress vector on an octahedral plane is then given by:
The normal component of the stress vector at point O associated with the octahedral plane is
which is the mean normal stress or hydrostatic stress. This value is the same in all eight octahedral planes. The shear stress on the octahedral plane is then
Analysis of stress
The analysis of stress within a body implies the determination at each point of the body of the magnitudes of the nine stress components. In other words, it is the determination of the internal distribution of stresses. A stress analysis is required in engineering, e.g., civil engineering and mechanical engineering, for the study and design of structures, e.g., tunnels, dams, mechanical parts, and structural frames among others, under prescribed or expected loads.
To determine the distribution of stress in the structure it is necessary to solve a boundary-value problem by specifying the boundary conditions, i.e. displacements and/or forces on the boundary. Constitutive equations, such as e.g. Hooke's Law for linear elastic materials, are used to describe the stress:strain relationship in these calculations. A boundary-value problem based on the theory of elasticity is applied to structures expected to deform elastically, i.e. infinitesimal strains, under design loads. When the loads applied to the structure induce plastic deformations, the theory of plasticity is implemented.
Approximate solutions for boundary-value problems can be obtained through the use numerical methods such as the Finite Element Method, the Finite Difference Method, and the Boundary Element Method, which are implemented in computer programs. Analytical or close-form solutions can be obtained for simple geometries, constitutive relations, and boundary conditions.
Alternatively, experimental determination of stresses can be carried out using the photoelastic method.
In design of structures, calculated stresses are restricted to be less than an specified allowable stress, also known as working or designed stress, that is chosen as some fraction of the yield strength or of the ultimate strength of the material which the structure is made of. The ratio of the ultimate stress to the allowable stress is defined as the factor of safety. Laboratory test are usually performed on material samples in order to determine the yield strength and the ultimate strength that the material can withstand before failure.
All real objects occupy a three-dimensional space. The stress analysis can be simplified in cases where the physical dimensions and the loading conditions allows the structure to be assumed as one-dimensional or two-dimensional. For a two-dimensional analysis a plane stress or a plane strain condition can be assumed.
Uniaxial stress
If two of the dimensions of the object are very large or very small compared to the others, the object may be modelled as one-dimensional. In this case the stress tensor has only one component and is indistinguishable from a scalar. One-dimensional objects include a piece of wire loaded at the ends and a metal sheet loaded on the face and viewed up close and through the cross section.
When a structural element is elongated or compressed, its cross-sectional area changes by an amount that depends on the Poisson's ratio of the material. In engineering applications, structural members experience small deformations and the reduction in cross-sectional area is very small and can be neglected, i.e., the cross-sectional area is assumed constant during deformation. For this case, the stress is called engineering stress or nominal stress. In some other cases, e.g., elastomers and plastic materials, the change in cross-sectional area is significant, and the stress must be calculated assuming the current cross-sectional area instead of the initial cross-sectional area. This is termed true stress and is expressed as
 ,
,
where
 is the nominal (engineering) strain, and
is the nominal (engineering) strain, and
 is nominal (engineering) stress.
is nominal (engineering) stress.
The relationship between true strain and engineering strain is given by
 .
.
In uniaxial tension, true stress is then greater than nominal stress. The converse holds in compression.

Plane stress
A state of plane stress exists when one of the three principal  , stresses is zero. This usually occurs in structural elements where one dimension is very small compared to the other two, i.e. the element is flat or thin. In this case, the stresses are negligible with respect to the smaller dimension as they are not able to develop within the material and are small compared to the in-plane stresses. Therefore, the face of the element is not acted by loads and the structural element can be analyzed as two-dimensional, e.g. thin-walled structures such as plates subject to in-plane loading or thin cylinders subject to pressure loading. The other three non-zero components remain constant over the thickness of the plate. The stress tensor can then be approximated by:
, stresses is zero. This usually occurs in structural elements where one dimension is very small compared to the other two, i.e. the element is flat or thin. In this case, the stresses are negligible with respect to the smaller dimension as they are not able to develop within the material and are small compared to the in-plane stresses. Therefore, the face of the element is not acted by loads and the structural element can be analyzed as two-dimensional, e.g. thin-walled structures such as plates subject to in-plane loading or thin cylinders subject to pressure loading. The other three non-zero components remain constant over the thickness of the plate. The stress tensor can then be approximated by:
 .
.
The corresponding strain tensor is:
in which the non-zero  term arises from the Poisson's effect. This strain term can be temporarily removed from the stress analysis to leave only the in-plane terms, effectively reducing the analysis to two dimensions.
term arises from the Poisson's effect. This strain term can be temporarily removed from the stress analysis to leave only the in-plane terms, effectively reducing the analysis to two dimensions.

Plane strain
If one dimension is very large compared to the others, the principal strain in the direction of the longest dimension is constrained and can be assumed as zero, yielding a plane strain condition (Figure 7.2). In this case, though all principal stresses are non-zero, the principal stress in the direction of the longest dimension can be disregarded for calculations. Thus, allowing a two dimensional analysis of stresses, e.g. a dam analyzed at a cross section loaded by the reservoir.
Stress transformation in plane stress and plane strain
Consider a point  in a continuum under a state of plane stress, or plane strain, with stress components
in a continuum under a state of plane stress, or plane strain, with stress components  and all other stress components equal to zero (Figure 7.1, Figure 8.1). From static equilibrium of an infinitesimal material element at
and all other stress components equal to zero (Figure 7.1, Figure 8.1). From static equilibrium of an infinitesimal material element at  (Figure 8.2), the normal stress
(Figure 8.2), the normal stress  and the shear stress
and the shear stress  on any plane perpendicular to the
on any plane perpendicular to the  -
- plane passing through
plane passing through  with a unit vector
with a unit vector  making an angle of
making an angle of  with the horizontal, i.e.
with the horizontal, i.e.  is the direction cosine in the
is the direction cosine in the  direction, is given by:
direction, is given by:
These equations indicate that in a plane stress or plane strain condition, one can determine the stress components at a point on all directions, i.e. as a function of  , if one knows the stress components
, if one knows the stress components  on any two perpendicular directions at that point. It is important to remember that we are considering a unit area of the infinitesimal element in the direction parallel to the
on any two perpendicular directions at that point. It is important to remember that we are considering a unit area of the infinitesimal element in the direction parallel to the  -
- plane.
plane.


The principal directions (Figure 8.3), i.e. orientation of the planes where the shear stress components are zero, can be obtained by making the previous equation for the shear stress  equal to zero. Thus we have:
equal to zero. Thus we have:
and we obtain
This equation defines two values  which are
which are  apart (Figure 8.3). The same result can be obtained by finding the angle
apart (Figure 8.3). The same result can be obtained by finding the angle  which makes the normal stress
which makes the normal stress  a maximum, i.e.
a maximum, i.e. 
The principal stresses  and
and  , or minimum and maximum normal stresses
, or minimum and maximum normal stresses  and
and  , respectively, can then be obtained by replacing both values of
, respectively, can then be obtained by replacing both values of  into the previous equation for
into the previous equation for  . This can be achieved by rearranging the equations for
. This can be achieved by rearranging the equations for  and
and  , first transposing the first term in the first equation and squaring both sides of each of the equations then adding them. Thus we have
, first transposing the first term in the first equation and squaring both sides of each of the equations then adding them. Thus we have
where
which is the equation of a circle of radius  centered at a point with coordinates
centered at a point with coordinates ![[\sigma_\mathrm{avg}, 0]\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/b6468372d603f706ec51b7825dc6e679.png) , called Mohr's circle. But knowing that for the principal stresses the shear stress
, called Mohr's circle. But knowing that for the principal stresses the shear stress  , then we obtain from this equation:
, then we obtain from this equation:
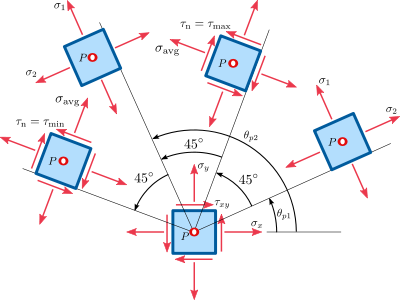
When  the infinitesimal element is oriented in the direction of the principal planes, thus the stresses acting on the rectangular element are principal stresses:
the infinitesimal element is oriented in the direction of the principal planes, thus the stresses acting on the rectangular element are principal stresses:  and
and  . Then the normal stress
. Then the normal stress  and shear stress
and shear stress  as a function of the principal stresses can be determined by making
as a function of the principal stresses can be determined by making  . Thus we have
. Thus we have
Then the maximum shear stress  occurs when
occurs when  , i.e.
, i.e.  (Figure 8.3):
(Figure 8.3):
Then the minimum shear stress  occurs when
occurs when  , i.e.
, i.e.  (Figure 8.3):
(Figure 8.3):
Graphical representation of stress at a point
Mohr's circle, Lame's stress ellipsoid (together with the stress director surface), and Cauchy's stress quadric are two-dimensional graphical representations of the state of stress at a point. They allow for the graphical determination of the magnitude of the stress tensor at a given point for all planes passing through that point. Mohr's circle is the most common graphical method.
Mohr's circle
Mohr's circle, named after Christian Otto Mohr, is the locus of points that represent the state of stress on individual planes at all their orientations. The abscissa,  , and ordinate,
, and ordinate,  , of each point on the circle are the normal stress and shear stress components, respectively, acting on a particular cut plane with a unit vector
, of each point on the circle are the normal stress and shear stress components, respectively, acting on a particular cut plane with a unit vector  with components
with components  .
.
Lame's stress ellipsoid
The surface of the ellipsoid represents the locus of the endpoints of all stress vectors acting on all planes passing through a given point in the continuum body. In other words, the endpoints of all stress vectors at a given point in the continuum body lie on the stress ellipsoid surface, i.e., the radius-vector from the center of the ellipsoid, located at the material point in consideration, to a point on the surface of the ellipsoid is equal to the stress vector on some plane passing through the point. In two dimensions, the surface is represented by an ellipse (Figure coming).
Cauchy's stress quadric
The Cauchy's stress quadric, also called the stress surface, is a surface of the second order that traces the variation of the normal stress vector  as the orientation of the planes passing through a given point is changed.
as the orientation of the planes passing through a given point is changed.
Graphical representation of the stress field
The complete state of stress in a body at a particular deformed configuration, i.e., at a particular time during the motion of the body, implies knowing the six independent components of the stress tensor  , or the three principal stresses
, or the three principal stresses  , at each material point in the body at that time. However, numerical analysis and analytical methods allow only for the calculation of the stress tensor at a certain number of discrete material points. To graphically represent in two dimensions this partial picture of the stress field different sets of contour lines can be used[19]:
, at each material point in the body at that time. However, numerical analysis and analytical methods allow only for the calculation of the stress tensor at a certain number of discrete material points. To graphically represent in two dimensions this partial picture of the stress field different sets of contour lines can be used[19]:
- Isobars are curves along which the principal stress, e.g.,
 is constant.
is constant. - Isochromatics are curves along which the maximum shear stress is constant. This curves are directly determined using photoelasticity methods.
- Isopachs are curves along which the mean normal stress is constant
- Isostatics or stress trajectories are a system of curves which are at each material point tangent to the principal axes of stress.
- Isoclinics are curves on which the principal axes make a constant angle with a given fixed reference direction. These curves can also be obtained directly by photoelasticity methods.
- Slip lines are curves on which the shear stress is a maximum.
Alternative measures of stress
The Cauchy stress tensor is not the only measure of stress that is used in practice. Other measures of stress include the first and second Piola-Kirchhoff stress tensors, the Biot stress tensor, and the Kirchhoff stress tensor.
Piola-Kirchhoff stress tensor
In the case of finite deformations, the Piola-Kirchhoff stress tensors are used to express the stress relative to the reference configuration. This is in contrast to the Cauchy stress tensor which expresses the stress relative to the present configuration. For infinitesimal deformations or rotations, the Cauchy and Piola-Kirchhoff tensors are identical. These tensors take their names from Gabrio Piola and Gustav Kirchhoff.
Whereas the Cauchy stress tensor,  relates stresses in the current configuration, the deformation gradient and strain tensors are described by relating the motion to the reference configuration; thus not all tensors describing the state of the material are in either the reference or current configuration. Having the stress, strain and deformation all described either in the reference or current configuration would make it easier to define constitutive models (for example, the Cauchy Stress tensor is variant to a pure rotation, while the deformation strain tensor is invariant; thus creating problems in defining a constitutive model that relates a varying tensor, in terms of an invariant one during pure rotation; as by definition constitutive models have to be invariant to pure rotations). The 1st Piola-Kirchhoff stress tensor,
relates stresses in the current configuration, the deformation gradient and strain tensors are described by relating the motion to the reference configuration; thus not all tensors describing the state of the material are in either the reference or current configuration. Having the stress, strain and deformation all described either in the reference or current configuration would make it easier to define constitutive models (for example, the Cauchy Stress tensor is variant to a pure rotation, while the deformation strain tensor is invariant; thus creating problems in defining a constitutive model that relates a varying tensor, in terms of an invariant one during pure rotation; as by definition constitutive models have to be invariant to pure rotations). The 1st Piola-Kirchhoff stress tensor,  is one possible solution to this problem. It defines a family of tensors, which describe the configuration of the body in either the current or the reference state.
is one possible solution to this problem. It defines a family of tensors, which describe the configuration of the body in either the current or the reference state.
The 1st Piola-Kirchhoff stress tensor,  relates forces in the present configuration with areas in the reference ("material") configuration.
relates forces in the present configuration with areas in the reference ("material") configuration.
where  is the deformation gradient and
is the deformation gradient and  is the Jacobian determinant.
is the Jacobian determinant.
In terms of components with respect to an orthonormal basis, the first Piola-Kirchhoff stress is given by
Because it relates different coordinate systems, the 1st Piola-Kirchhoff stress is a two-point tensor. In general, it is not symmetric. The 1st Piola-Kirchhoff stress is the 3D generalization of the 1D concept of engineering stress.
If the material rotates without a change in stress state (rigid rotation), the components of the 1st Piola-Kirchhoff stress tensor will vary with material orientation.
The 1st Piola-Kirchhoff stress is energy conjugate to the deformation gradient.
2nd Piola-Kirchhoff stress tensor
Whereas the 1st Piola-Kirchhoff stress relates forces in the current configuration to areas in the reference configuration, the 2nd Piola-Kirchhoff stress tensor  relates forces in the reference configuration to areas in the reference configuration. The force in the reference configuration is obtained via a mapping that preserves the relative relationship between the force direction and the area normal in the current configuration.
relates forces in the reference configuration to areas in the reference configuration. The force in the reference configuration is obtained via a mapping that preserves the relative relationship between the force direction and the area normal in the current configuration.
In index notation with respect to an orthonormal basis,
This tensor is symmetric.
If the material rotates without a change in stress state (rigid rotation), the components of the 2nd Piola-Kirchhoff stress tensor will remain constant, irrespective of material orientation.
The 2nd Piola-Kirchhoff stress tensor is energy conjugate to the Green-Lagrange finite strain tensor.
See also
- Bending
- Linear elasticity
- Kelvin probe force microscope
- Residual stress
- Shot peening
- Strain
- Strain tensor
- Stress-energy tensor
- Stress-strain curve
- Stress concentration
- Virial stress
- Von Mises stress
- Yield stress
- Yield surface
References
- ↑ Walter D. Pilkey, Orrin H. Pilkey (1974). Mechanics of solids. p. 292. http://books.google.com/books?id=d7I8AAAAIAAJ&q=average+shear+stress+approximation&dq=average+shear+stress+approximation&ei=FdBkS837NJPyNMLQzNgB&cd=2.
- ↑ 2.0 2.1 2.2 2.3 Chen
- ↑ 3.0 3.1 Smith & Truesdell p.97
- ↑ Slaughter
- ↑ 5.0 5.1 Lubliner
- ↑ 6.0 6.1 6.2 6.3 Liu
- ↑ 7.0 7.1 7.2 Wu
- ↑ 8.0 8.1 8.2 8.3 Fung
- ↑ 9.0 9.1 9.2 Mase
- ↑ 10.0 10.1 10.2 10.3 Atanackovic
- ↑ 11.0 11.1 11.2 11.3 11.4 Irgens
- ↑ 12.0 12.1 12.2 12.3 Chadwick
- ↑ Maxwell pointed out that nonvanishing body moments exist in a magnet in a magnetic field and in a dielectric material in an electric field with different planes of polarization. Fung p.76.
- ↑ Couple stresses and body couples were first explored by Voigt and Cosserat, and later reintroduced by Mindlin in 1960 on his work for Bell Labs on pure quartz crystals. Richards p.55.
- ↑ Truesdell and Topin 1960
- ↑ Basar
- ↑ Hjelmstad
- ↑ Chatterjee
- ↑ 19.0 19.1 Jaeger
- ↑ Ameen
- ↑ Prager
Bibliography
- Ameen, Mohammed (2005). Computational elasticity: theory of elasticity and finite and boundary element methods. Alpha Science Int'l Ltd.. pp. 33–66. ISBN 184265201X. http://books.google.ca/books?id=Gl9cFyLrdrcC&lpg=PP1&pg=PA33#v=onepage&q=&f=false.
- Atanackovic, Teodor M.; Guran, Ardéshir (2000). Theory of elasticity for scientists and engineers. Springer. pp. 1–46. ISBN 081764072X. http://books.google.ca/books?id=uQrBWdcGmjUC&lpg=PP1&pg=PA1#v=onepage&q=&f=false.
- Beer, Ferdinand Pierre; Elwood Russell Johnston, John T. DeWolf (1992). Mechanics of Materials. McGraw-Hill Professional. ISBN 0071129391.
- Brady, B.H.G.; E.T. Brown (1993). Rock Mechanics For Underground Mining (Third ed.). Kluwer Academic Publisher. pp. 17–29. ISBN 0412475502. http://books.google.ca/books?id=s0BaKxL11KsC&lpg=PP1&pg=PA18#v=onepage&q=&f=false.
- Chadwick, Peter (1999). Continuum mechanics: concise theory and problems. Dover books on physics (2 ed.). Dover Publications. pp. 90–106. ISBN 0486401804. http://books.google.ca/books?id=QSXIHQsus6UC&lpg=PA1&pg=PA95#v=onepage&q=&f=false.
- Chakrabarty, J. (2006). Theory of plasticity (3 ed.). Butterworth-Heinemann. pp. 17–32. ISBN 0750666382. http://books.google.ca/books?id=9CZsqgsfwEAC&lpg=PP1&dq=related%3AISBN0486435946&lr=&rview=1&pg=PA17#v=onepage&q=&f=false.
- Chatterjee, Rabindranath (1999). Mathematical Theory of Continuum Mechanics. Alpha Science Int'l Ltd.. pp. 111–157. ISBN 8173192448. http://books.google.com/books?id=v2F84PwH0BkC&lpg=PP1&pg=PA111#v=onepage&q=&f=false.
- Chen, Wai-Fah; Han, Da-Jian (2007). Plasticity for structural engineers. J. Ross Publishing. pp. 46–71. ISBN 1932159754. http://books.google.com/books?id=E8jptvNgADYC&lpg=PP1&pg=PA46#v=onepage&q=&f=false.
- Chou, Pei Chi; Pagano, N.J. (1992). Elasticity: tensor, dyadic, and engineering approaches. Dover books on engineering. Dover Publications. pp. 1–33. ISBN 0486669580. http://books.google.com/books?id=9-pJ7Kg5XmAC&lpg=PP1&pg=PA1#v=onepage&q=&f=false.
- Davis, R. O.; Selvadurai. A. P. S. (1996). Elasticity and geomechanics. Cambridge University Press. pp. 16–26. ISBN 0521498279. http://books.google.ca/books?id=4Z11rZaUn1UC&lpg=PP1&pg=PA16#v=onepage&q=&f=false.
- Dieter, G. E. (3 ed.). (1989). Mechanical Metallurgy. New York: McGraw-Hill. ISBN 0-07-100406-8.
- Fung, Yuan-cheng; Tong, Pin (2001). Classical and computational solid mechanics. Volume 1 of Advanced series in engineering science. World Scientific. pp. 66–96. ISBN 9810241240. http://books.google.ca/books?id=hmyiIiiv4FUC&lpg=PP1&pg=PA66#v=onepage&q=&f=false.
- Hjelmstad, Keith D. (2005). Fundamentals of structural mechanics. Prentice-Hall international series in civil engineering and engineering mechanics (2 ed.). Springer. pp. 103–130. ISBN 038723330X. http://books.google.ca/books?id=LVTYjmcdvPwC&lpg=PP1&pg=PA103#v=onepage&q=&f=false.
- Holtz, Robert D.; Kovacs, William D. (1981). An introduction to geotechnical engineering. Prentice-Hall civil engineering and engineering mechanics series. Prentice-Hall. ISBN 0134843940. http://books.google.ca/books?id=yYkYAQAAIAAJ&dq=inauthor:%22William+D.+Kovacs%22&ei=kF-MS5LRKpfCM9vEhIYN&cd=1.
- Irgens, Fridtjov (2008). Continuum mechanics. Springer. pp. 42–81. ISBN 3540742972. http://books.google.com/books?id=q5dB7Gf4bIoC&lpg=PA46&dq=cauchy's%20fundamental%20lemma&pg=PA46#v=onepage&q=&f=false.
- Jaeger, John Conrad; Cook, N.G.W, & Zimmerman, R.W. (2007). Fundamentals of rock mechanics (Fourth ed.). Wiley-Blackwell. pp. 9–41. ISBN 0632057599. http://books.google.com/books?id=FqADDkunVNAC&lpg=PP1&pg=PA10#v=onepage&q=&f=false.
- Jones, Robert Millard (2008). Deformation Theory of Plasticity. Bull Ridge Corporation. pp. 95–112. ISBN 0978722310. http://books.google.ca/books?id=kiCVc3AJhVwC&lpg=PP1&pg=PA95#v=onepage&q=&f=false.
- Jumikis, Alfreds R. (1969). Theoretical soil mechanics: with practical applications to soil mechanics and foundation engineering. Van Nostrand Reinhold Co.. ISBN 0442041993. http://books.google.ca/books?id=NPZRAAAAMAAJ&source=gbs_navlinks_s.
- Landau, L.D. and E.M.Lifshitz. (1959). Theory of Elasticity.
- Love, A. E. H. (4 ed.). (1944). Treatise on the Mathematical Theory of Elasticity. New York: Dover Publications. ISBN 0-486-60174-9.
- Liu, I-Shih (2002). Continuum mechanics. Springer. pp. 41–50. ISBN 3540430199. http://books.google.com/books?id=-gWqM4uMV6wC&lpg=PA45&dq=cauchy's%20fundamental%20lemma&pg=PA43#v=onepage&q=&f=false.
- Lubliner, Jacob (2008). Plasticity Theory (Revised Edition). Dover Publications. ISBN 0486462900. http://www.ce.berkeley.edu/~coby/plas/pdf/book.pdf.
- Marsden, J. E.; Hughes, T. J. R. (1994). Mathematical Foundations of Elasticity. Dover Publications. pp. 132–142. ISBN 0486678652. http://books.google.ca/books?id=RjzhDL5rLSoC&lpg=PR1&pg=PA133#v=onepage&q&f=false.
- Mase, George E. (1970). Continuum Mechanics. McGraw-Hill. pp. 44–76. ISBN 0070406634. http://books.google.ca/books?id=bAdg6yxC0xUC&rview=1.
- Mase, G. Thomas; George E. Mase (1999). Continuum Mechanics for Engineers (Second ed.). CRC Press. pp. 47–102. ISBN 0-8493-1855-6. http://books.google.ca/books?id=uI1ll0A8B_UC&lpg=PP1&rview=1&pg=PA47#v=onepage&q=&f=false.
- Parry, Richard Hawley Grey (2004). Mohr circles, stress paths and geotechnics (2 ed.). Taylor & Francis. pp. 1–30. ISBN 0415272971. http://books.google.ca/books?id=u_rec9uQnLcC&lpg=PP1&dq=mohr%20circles%2C%20sterss%20paths%20and%20geotechnics&pg=PA1#v=onepage&q=&f=false.
- Prager, William (2004). Introduction to mechanics of continua. Dover Publications. pp. 43–61. ISBN 0486438090. http://books.google.ca/books?id=Feer6-hn9zsC&lpg=PP1&rview=1&pg=PA43#v=onepage&q=&f=false.
- Rees, David (2006). Basic Engineering Plasticity - An Introduction with Engineering and Manufacturing Applications. Butterworth-Heinemann. pp. 1–32. ISBN 0750680253. http://books.google.ca/books?id=4KWbmn_1hcYC&lpg=PP1&pg=PA1#v=onepage&q=&f=false.
- Smith, Donald Ray; Truesdell, Clifford (1993). An introduction to continuum mechanics -after Truesdell and Noll. Springer. ISBN 0792324544. http://books.google.com/books?id=ZcWC7YVdb4wC&lpg=PP1&pg=PA97#v=onepage&q&f=false.
- Timoshenko, Stephen P.; James Norman Goodier (1970). Theory of Elasticity (Third ed.). McGraw-Hill International Editions. ISBN 0-07-085805-5.
- Timoshenko, Stephen P. (1983). History of strength of materials: with a brief account of the history of theory of elasticity and theory of structures. Dover Books on Physics. Dover Publications. ISBN 0486611876.
- Wu, Han-Chin (2005). Continuum mechanics and plasticity. CRC Press. pp. 45–78. ISBN 1584883634. http://books.google.com/books?id=OS4mICsHG3sC&lpg=PP1&pg=PA45#v=onepage&q=&f=false.


![\boldsymbol{\sigma}=
\left[{\begin{matrix}
\sigma _{11} & \sigma _{12} & \sigma _{13} \\
\sigma _{21} & \sigma _{22} & \sigma _{23} \\
\sigma _{31} & \sigma _{32} & \sigma _{33} \\
\end{matrix}}\right]
\equiv \left[{\begin{matrix}
\sigma _{xx} & \sigma _{xy} & \sigma _{xz} \\
\sigma _{yx} & \sigma _{yy} & \sigma _{yz} \\
\sigma _{zx} & \sigma _{zy} & \sigma _{zz} \\
\end{matrix}}\right]
\equiv \left[{\begin{matrix}
\sigma _x & \tau _{xy} & \tau _{xz} \\
\tau _{yx} & \sigma _y & \tau _{yz} \\
\tau _{zx} & \tau _{zy} & \sigma _z \\
\end{matrix}}\right]
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/881f8450625c6b193d2cbf8ad3bff9e6.png)
























![\boldsymbol{\sigma}= \sigma_{ij} = \left[{\begin{matrix} \mathbf{T}^{(\mathbf{e}_1)} \\
\mathbf{T}^{(\mathbf{e}_2)} \\
\mathbf{T}^{(\mathbf{e}_3)} \\
\end{matrix}}\right] =
\left[{\begin{matrix}
\sigma _{11} & \sigma _{12} & \sigma _{13} \\
\sigma _{21} & \sigma _{22} & \sigma _{23} \\
\sigma _{31} & \sigma _{32} & \sigma _{33} \\
\end{matrix}}\right] \equiv \left[{\begin{matrix}
\sigma _{xx} & \sigma _{xy} & \sigma _{xz} \\
\sigma _{yx} & \sigma _{yy} & \sigma _{yz} \\
\sigma _{zx} & \sigma _{zy} & \sigma _{zz} \\
\end{matrix}}\right] \equiv \left[{\begin{matrix}
\sigma _x & \tau _{xy} & \tau _{xz} \\
\tau _{yx} & \sigma _y & \tau _{yz} \\
\tau _{zx} & \tau _{zy} & \sigma _z \\
\end{matrix}}\right]
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/c168045706a6fe957d114cb69e958a66.png)



![\left[{\begin{matrix}
T^{(n)}_1 & T^{(n)}_2 & T^{(n)}_3\end{matrix}}\right]=\left[{\begin{matrix}
n_1 & n_2 & n_3
\end{matrix}}\right]\cdot
\left[{\begin{matrix}
\sigma _{11} & \sigma _{12} & \sigma _{13} \\
\sigma _{21} & \sigma _{22} & \sigma _{23} \\
\sigma _{31} & \sigma _{32} & \sigma _{33} \\
\end{matrix}}\right]
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/4d9b6ebdbf17ae3dde91b58f6f102e4d.png)

![\left[{\begin{matrix}
\sigma^'_{11} & \sigma^'_{12} & \sigma^'_{13} \\
\sigma^'_{21} & \sigma^'_{22} & \sigma^'_{23} \\
\sigma^'_{31} & \sigma^'_{32} & \sigma^'_{33} \\
\end{matrix}}\right]=\left[{\begin{matrix}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33} \\
\end{matrix}}\right]\left[{\begin{matrix}
\sigma_{11} & \sigma_{12} & \sigma_{13} \\
\sigma_{21} & \sigma_{22} & \sigma_{23} \\
\sigma_{31} & \sigma_{32} & \sigma_{33} \\
\end{matrix}}\right]\left[{\begin{matrix}
a_{11} & a_{21} & a_{31} \\
a_{12} & a_{22} & a_{32} \\
a_{13} & a_{23} & a_{33} \\
\end{matrix}}\right]
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/b97f38379ba30fcabc294211e2874e88.png)





























![\begin{align}
\tau_\mathrm{n}^2 &= \left( T^{(n)} \right)^2-\sigma_\mathrm{n}^2 \\
&=\sigma_1^2n_1^2+\sigma_2^2n_2^2+\sigma_3^2n_3^2-\left(\sigma_1n_1^2+\sigma_2n_2^2+\sigma_3n_3^2\right)^2 \\
&=(\sigma_1^2-\sigma_2^2)n_1^2+(\sigma_2^2-\sigma_3^2)n_2^2+\sigma_3^2-\left[\left(\sigma_1-\sigma_3\right)n_1^2+\left(\sigma_2-\sigma_2\right)n_2^2+\sigma_3\right]^2 \\
&= (\sigma_1-\sigma_2)^2n_1^2n_2^2+(\sigma_2-\sigma_3)^2n_2^2n_3^2+(\sigma_1-\sigma_3)^2n_1^2n_3^2 \\
\end{align}
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/76244d057842e43bbb572186b05deec7.png)









![\begin{align}
\left[n_1^2\sigma_1^2+n_2^2\sigma_2^2+n_3^2\sigma_3^2\right]-2\left(\sigma_1n_1^2+\sigma_2n_2^2+\sigma_3n_3^2\right)\sigma_\mathrm{n}+\lambda\left(n_1^2+n_2^2+n_3^2\right)&=0 \\
\left[\tau_\mathrm{n}^2+\left(\sigma_1n_1^2+\sigma_2n_2^2+\sigma_3n_3^2\right)^2\right]-2\sigma_\mathrm{n}^2+\lambda&=0 \\
\left[\tau_\mathrm{n}^2+\sigma_\mathrm{n}^2\right]-2\sigma_\mathrm{n}^2+\lambda &=0 \\
\lambda &= \sigma_\mathrm{n}^2-\tau_\mathrm{n}^2 \\
\end{align}\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/fe45d449180531ff7338ae1d22f3b8f3.png)
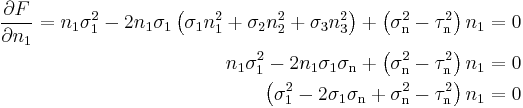


















![\begin{align}
\ s_{ij} &= \sigma_{ij} - \frac{\sigma_{kk}}{3}\delta_{ij} \\
\left[{\begin{matrix}
s_{11} & s_{12} & s_{13} \\
s_{21} & s_{22} & s_{23} \\
s_{31} & s_{32} & s_{33} \\
\end{matrix}}\right]
&=\left[{\begin{matrix}
\sigma_{11} & \sigma_{12} & \sigma_{13} \\
\sigma_{21} & \sigma_{22} & \sigma_{23} \\
\sigma_{31} & \sigma_{32} & \sigma_{33} \\
\end{matrix}}\right]-\left[{\begin{matrix}
p & 0 & 0 \\
0 & p & 0 \\
0 & 0 & p \\
\end{matrix}}\right] \\
&=\left[{\begin{matrix}
\sigma_{11}-p & \sigma_{12} & \sigma_{13} \\
\sigma_{21} & \sigma_{22}-p & \sigma_{23} \\
\sigma_{31} & \sigma_{32} & \sigma_{33}-p \\
\end{matrix}}\right] \\
\end{align}\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/5d94556a2dd1d22154b29fd131ee8f45.png)


![\begin{align}
J_2 &= \textstyle{\frac{1}{2}}s_{ij}s_{ji} \\
&= -s_1s_2 - s_2s_3 - s_3s_1 \\
&= \tfrac{1}{6}\left[(\sigma_{11} - \sigma_{22})^2 + (\sigma_{22} - \sigma_{33})^2 + (\sigma_{33} - \sigma_{11})^2 \right ] + \sigma_{12}^2 + \sigma_{23}^2 + \sigma_{31}^2 \\
&= \tfrac{1}{6}\left[(\sigma_1 - \sigma_2)^2 + (\sigma_2 - \sigma_3)^2 + (\sigma_3 - \sigma_1)^2 \right ] \\
&= \tfrac{1}{3}I_1^2-I_2\\
J_3 &= \det(s_{ij}) \\
&= \tfrac{1}{3}s_{ij}s_{jk}s_{ki} \\
&= s_1s_2s_3 \\
&= \tfrac{2}{27}I_1^3 - \tfrac{1}{3}I_1 I_2 + I_3
\end{align}
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/3376a9131e722a3f7beb2266bc4f5f44.png)
![\sigma_\mathrm e = \sqrt{3~J_2} = \sqrt{\tfrac{1}{2}~\left[(\sigma_1-\sigma_2)^2 + (\sigma_2-\sigma_3)^2 + (\sigma_3-\sigma_1)^2 \right]}
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/aee2f84fcb3bb0d5e6e32957f509aef0.png)


![\begin{align}
\tau_\mathrm{oct} &=\sqrt{T_i^{(n)}T_i^{(n)}-\sigma_\mathrm{n}^2} \\
&=\left[\tfrac{1}{3}(\sigma_1^2+\sigma_2^2+\sigma_3^2)-\tfrac{1}{9}(\sigma_1+\sigma_2+\sigma_3)^2\right]^{1/2} \\
&=\tfrac{1}{3}\left[(\sigma_1-\sigma_2)^2+(\sigma_2-\sigma_3)^2+(\sigma_3-\sigma_1)^2\right]^{1/2} = \tfrac{1}{3}\sqrt{2I_1^2-6I_2} = \sqrt{\tfrac{2}{3}J_2}
\end{align}
\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/fae8bfa5e4e546ff32b29860778b4987.png)





![\begin{align}
\left[ \sigma_\mathrm{n} - \tfrac{1}{2} ( \sigma_x + \sigma_y )\right]^2 + \tau_\mathrm{n}^2 &= \left[\tfrac{1}{2}(\sigma_x - \sigma_y)\right]^2 + \tau_{xy}^2 \\
(\sigma_\mathrm{n} - \sigma_\mathrm{avg})^2 + \tau_\mathrm{n}^2 &= R^2 \end{align}\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/129d8cd805c51a932abec44d9278cf67.png)
![R = \sqrt{\left[\tfrac{1}{2}(\sigma_x - \sigma_y)\right]^2 + \tau_{xy}^2} \quad \text{and} \quad \sigma_\mathrm{avg} = \tfrac{1}{2} ( \sigma_x + \sigma_y )\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/1ec1e07d2cdac0309f69195afd137b6d.png)
![\sigma_1 =\sigma_\mathrm{max} = \tfrac{1}{2}(\sigma_x + \sigma_y) + \sqrt{\left[\tfrac{1}{2}(\sigma_x - \sigma_y)\right]^2 + \tau_{xy}^2}\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/d250eba2c2754c819f4b94feb4d82c92.png)
![\sigma_2 =\sigma_\mathrm{min} = \tfrac{1}{2}(\sigma_x + \sigma_y) - \sqrt{\left[\tfrac{1}{2}(\sigma_x - \sigma_y)\right]^2 + \tau_{xy}^2}\,\!](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/582fb5788adbde36223356dbff238e18.png)